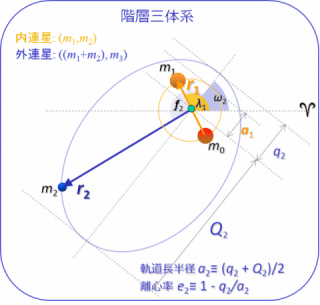
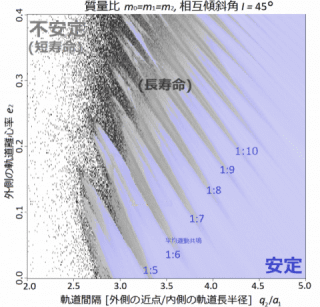
階層三体問題
感染症数理モデル

論文
Masaya M. Saito ,Kotoe Katayama,Akira Naruse,Peiying Ruan,Michio Murakami,Tomoaki Okuda,Tetsuo Ysutaka,Wataru Naito,Masaharu Tsubokura,Seiya Imoto.
Effects of inbound attendees of a mass gathering event on the COVID-19 epidemic using individual-based simulations. PLOS ONE 20(4), e0321288, 2025. Journal Site
M.M. Saito, K. Tanikawa State Transitions in Hieratical Triple System and Its Detection. Journal of Advances in Artificial Life Robotics 3(1), 1-7, 2022. Journal Site
Masaya M. Saito, Nobuo Hirotsu, Hiroka Hamada, Mio Takei, Keisuke Honda, Takamichi Baba, Takahiro Hasegawa, Yoshitake Kitanishi. Reconstructing the household transmission of influenza in the suburbs of Tokyo based on clinical cases. Theoretical Biology and Medical Modelling (2021) 18:7, 1-10, 2021.
M. M. Saito, H. Nishiura, T. Higuchi, Reconstructing the transmission dynamics of rubella in Japan, 2012-2013. PLoS ONE 13(10), 1-15, 2018 Journal Site
M. M. Saito, K. Ejima, R. Kinoshita, H. Nishiura, Assessing the effectiveness and cost-benefit of test-and-vaccinate policy for supplementary vaccination against rubella with limited doses. International Journal of Environmental Research and Public Health 15, 572, 1-12, 2018.
Suzuki, M.M. Saito, Y. Hayashi, M. Saito, T. Yajima, N. Sano, M. Tanaka, T. Suzuki, Statistical analysis of influenza propagation pattern using prescription data from Tochigi Prefecture, Total Quality Science 3(1), 35-45, 2017.
H. Nishiura, A. Endo, M. Saitoh, R. Kinoshita, R. Ueno, S. Nakaoka, Y. Miyamatsu, Y. Dong, G. Chowell, K. Mizumoto, Identifying determinants of heterogeneous transmission dynamics of the Middle East respiratory syndrome (MERS) outbreak in the Republic of Korea, 2015: a retrospective epidemiological analysis. BMJ Open 6, 1-10, 2016.
K. Mizumoto, A. Endo, G. Chowell, Y. Miyamatsu, M. Saitoh, Real-time characterization of risks of death associated with the Middle East respiratory syndrome (MERS) in the Republic of Korea. BMC Medicine 13:228, 2015.
Masaya M. Saito, Seiya Imoto, Rui Yamaguchi, Masaharu Tsubokura, Masahiro Kami, Haruka Nakada, Hiroki Sato, Satoru Miyano, Tomoyuki Higuchi, Enhancement of Collective Immunity in Tokyo Metropolitan Area by Selective Vaccination against an Emerging Influenza Pandemic, PLoS ONE, 8(9), 2013: e72866. doi:10.1371/journal.pone.0072866, available at Journal-Site.
Masaya M. Saito, Seiya Imoto, Rui Yamaguchi, Masahiro Kami, Haruka Nakada, Hiroki Sato, Satoru Miyano, Tomoyuki Higuchi, Extension and verification of the SEIR model on the 2009 influenza A (H1N1) pandemic in Japan, Mathematical Biosciences, 246 (1), pp. 47-54, 2013, available at Journal-Site.
M. M. Saito and K. Tanikawa, `Disintegration process of hierarchical triple systems II: non-small mass third body orbiting equal-mass binary, Springer, 116, pp.1-10, 2013, available at Journal-Site.
M. M. Saito and K. Tanikawa, `Disintegration process of Hierarchical Triple Systems: Small-mass third body orbiting equal-mass binary', Celestial Mechanics and Dynamical Astronomy, Springer, 112, pp.235-251, 2012, available at Journal-Site.
M. M. Saito and K. Tanikawa, `The Rectilinear Three-body Problem using Symbol Sequence III. Non-Schubart Periodic Orbits', Celestial Mechanics and Dynamical Astronomy, Springer, 107, pp.397-407, 2010.
M. M. Saito and K. Tanikawa, `The Rectilinear Three-body Problem using Symbol Sequence II. Role of the periodic orbits', Celestial Mechanics and Dynamical Astronomy, Springer, 103, pp.191-207, 2009.
M. M. Saito and K. Tanikawa, `The Rectilinear Three-body Problem using Symbol Sequence I. Role of triple collision', Celestial Mechanics and Dynamical Astronomy, Springer, 98, pp.95-120, 2007.
V.V. Orlov, A.V. Petrova, K. Tanikawa, M.M. Saito, A.I. Martynova, `The Rectilinear Three-Body Problem', Celestial Mechanics and Dynamical Astronomy, Springer, 100, pp.93-120, 2008.
| もっと見る |
ブログ
Powershellの備忘録
- 出力をShift_JIS にする.
-
$PSDefaultParameterValues['*:Encoding'] ='oem'と書く. - 参考: utf 8 - Changing PowerShell's default output encoding to UTF-8 - Stack Overflow
-
Files
test.html (2021-07-28 ・ 0KB) |
505-11-10-公開講座-2・3体問題.pdf (2023-11-10 ・ 3231KB) |
fourier_comp.pdf (2023-08-01 ・ 293KB) |
least_sq.pdf (2021-08-17 ・ 75KB) |
how_to_use_smlnj_nlffi (2021-08-17 ・ 22KB) |
nlffi_st.zip (2021-08-20 ・ 24KB) |